Optimizing Traffic Flow: Balancing Speed and Safety on Highways
Written on
Chapter 1: The Autobahn Debate
As someone from Germany, I've often been questioned about the renowned Autobahn. This has led to numerous discussions about the (often debated) absence of speed limits on certain roads. I’ve spent endless hours weighing the pros and cons of having speed limits on highways. While I largely support the idea of speed regulations, today’s discussion will not center on that topic.
This exploration will not delve into whether unrestricted speed is advisable or if driving slower results in fewer accidents. Instead, it focuses on how we can optimize traffic flow, maximizing the number of vehicles on increasingly congested roadways.
A widespread belief suggests that higher speeds allow for more cars to fit on the road, thereby enhancing traffic flow. This notion parallels the operation of railroads, where faster trains can transport more cars from one point to another in the same timeframe. However, the dynamics of car traffic are not so straightforward. Let's investigate this further.
Section 1.1: Understanding Traffic Dynamics
The speed of a vehicle is defined by the distance it covers divided by the time taken.

In this equation, we also need to account for the time interval between vehicles on the highway. This requires considering both the distance traveled and the length of the vehicle itself.
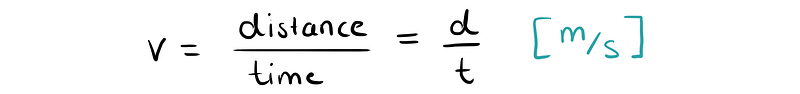
Let’s denote the length of a car as ( d_1 ) and the distance traveled as ( d_2 ). The total distance ( d ) between two vehicles can be expressed as the sum of these two variables. This relationship helps us determine the time gap between successive vehicles.
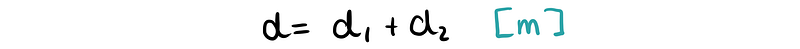
We can express traffic flow ( F(v) ), measured in cars per second, as a function of vehicle speed. In an hour, there are 3600 seconds, allowing us to establish the traffic flow ( F(v) ):
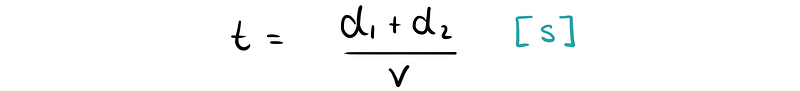
By factoring in the time interval between vehicles, which depends on both the vehicle length and the distance traveled, we arrive at a more nuanced equation for traffic flow.
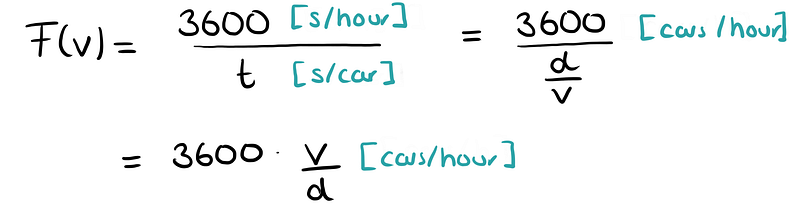
At first glance, it appears that to optimize traffic flow, one could simply reduce the distance between cars or increase their speed. However, this is a simplistic view, as driving faster necessitates maintaining a greater distance from the vehicle ahead for safety.
Section 1.2: The Importance of Braking Distance
To create a more realistic model, we must incorporate braking distance into our calculations. This involves two key factors: the reaction time to recognize the need to brake and the time taken to come to a complete stop.
Typically, it is assumed that a driver requires about one second to react to a braking scenario. During this second, a vehicle traveling at speed ( v ) covers a distance of ( v ) meters.
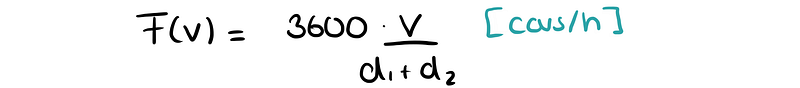
Next, we must determine how far a vehicle travels while decelerating to a stop. Using the formula for acceleration, we can quantify this distance:
Combining these distances gives us a full picture of how far a vehicle travels during the braking process.
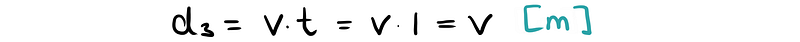
Thus, the traffic flow can be further refined with these variables taken into account.
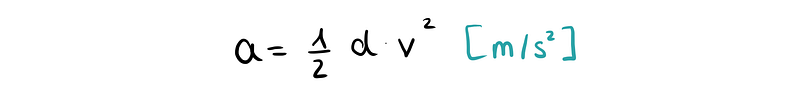
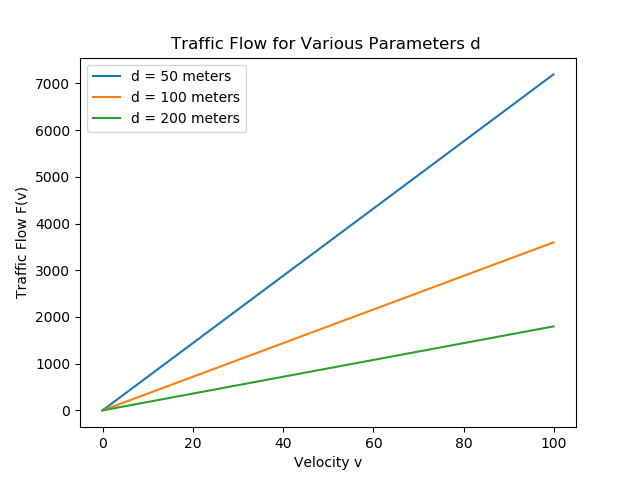
Let’s visualize this using various car lengths and a deceleration rate of 10 m/s².
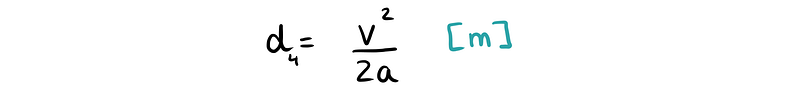
The resulting curve indicates an optimal point for traffic flow, which can be calculated mathematically by determining the derivative.
Chapter 2: The Realities of Highway Driving
As we analyze the data, we find that the optimal speed for maintaining traffic flow can be derived from the equation, yielding surprising results. For instance, if the average car length is 5 meters, the ideal speed to maximize traffic flow would be around 36 km/h (22 mph). For larger vehicles, such as trucks with an average length of 20 meters, this speed increases to about 72 km/h (44 mph).
However, this theoretical model may not reflect real-world conditions accurately. Drivers often do not maintain significant gaps between vehicles, which can lead to unpredictable situations on the road.
Section 2.1: The 2-Second Rule
In practice, maintaining a distance based solely on speed may not be necessary. The recommended approach is to keep a distance that allows for a two-second gap behind the vehicle in front. This rule simplifies our calculations and promotes safer driving practices.
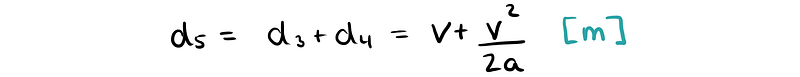
This adjustment leads to a clearer formula for traffic flow, as we can see from the updated calculations.
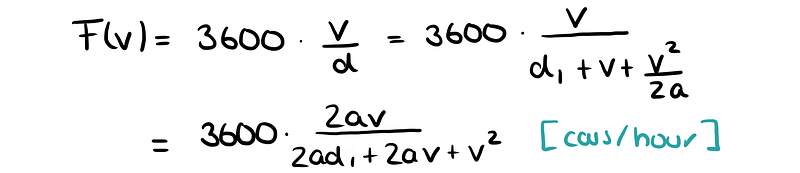
Examining the graphs, we observe that while the traffic flow increases with speed, it ultimately plateaus at around 1800 cars per hour, regardless of how fast vehicles are traveling.
Section 2.2: Conclusion and Safety Considerations
In conclusion, while there are many variables at play in highway traffic dynamics, the notion that speeding will always enhance traffic flow is misleading. Research indicates that sudden acceleration and braking can lead to traffic congestion and accidents. Therefore, it's crucial to prioritize safety and responsible driving over speed.
So, to enhance traffic flow, simply driving faster is not the panacea many believe it to be.
A final note: always prioritize safety on the roads. Avoid unnecessary speeding, especially in areas where it is hazardous. While some stretches of road may allow for high speeds, ensure that you are driving within safe limits.